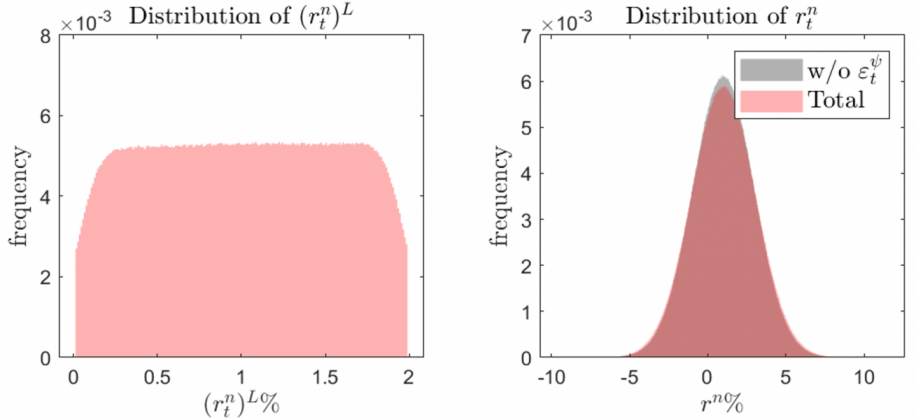
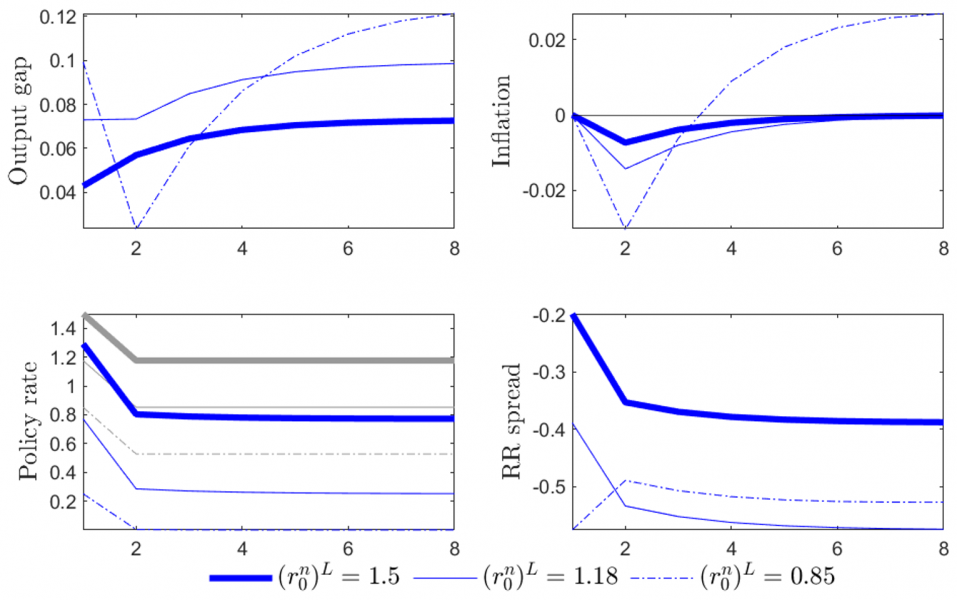
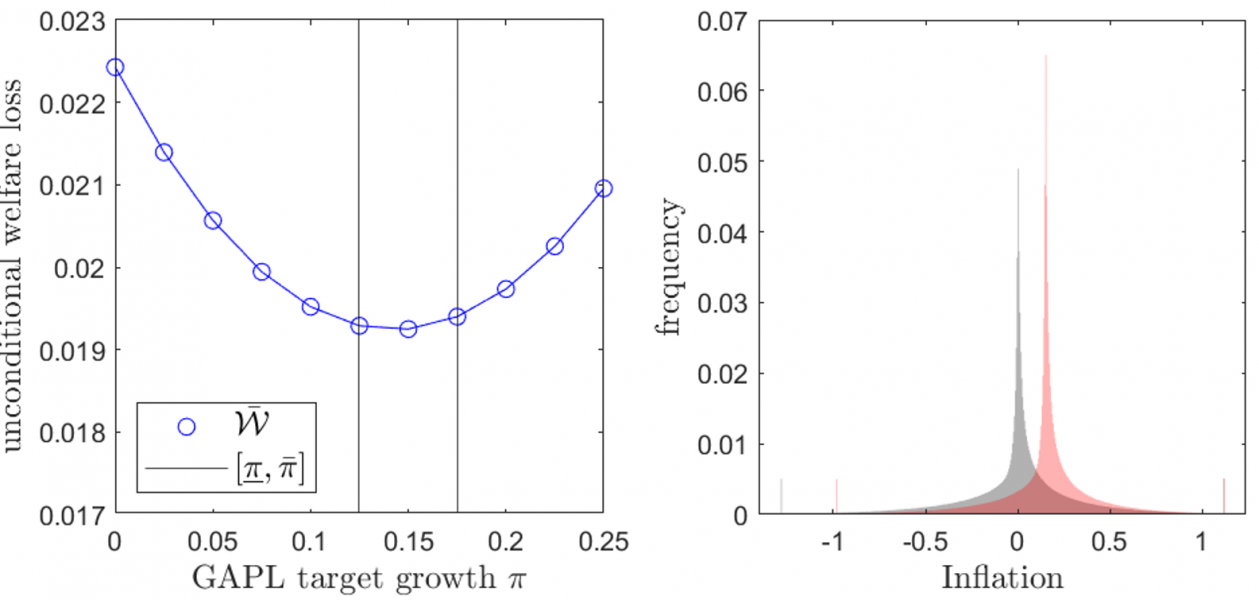
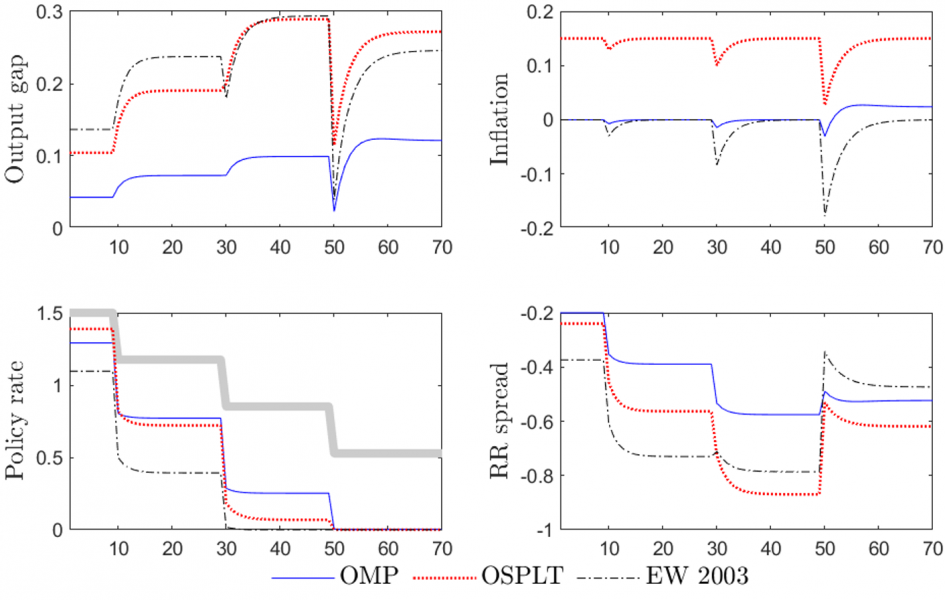
References
Daudignon, S., & Tristani, O. (2023). Monetary policy and the drifting natural rate of interest. ECB Working Paper.
Del Negro, M., Giannone, D., Giannoni, M. P., & Tambalotti, A. (2017). Safety, liquidity, and the natural rate of interest. Brookings Papers on Economic Activity, 2017(1), 235-316.
Eggertsson, G. B., & Woodford, M. (2003). Zero bound on interest rates and optimal monetary policy. Brookings papers on economic activity, 2003(1), 139-233.
Fiorentini, G., Galesi, A., Perez-Quiros, G., & Sentana, E. (2018). The rise and fall of the natural interest rate. Banco de Espana Working Paper 1822.
Fisher, J. D. (2015). On the Structural Interpretation of the Smets–Wouters “Risk Premium” Shock. Journal of Money, Credit and Banking, 47(2-3), 511-516.
Holston, K., Laubach, T., & Williams, J. C. (2017). Measuring the natural rate of interest: International trends and determinants. Journal of International Economics, 108, S59-S75.
Krishnamurthy, A., & Vissing-Jorgensen, A. (2012). The aggregate demand for treasury debt. Journal of Political Economy, 120(2), 233-267.
Laubach, T., & Williams, J. C. (2003). Measuring the natural rate of interest. Review of Economics and Statistics, 85(4), 1063-1070.
Michaillat, P., & Saez, E. (2021). Resolving New Keynesian anomalies with wealth in the utility function. Review of Economics and Statistics, 103(2), 197-215.
Platzer, J., & Peruffo, M. (2022). Secular Drivers of the Natural Rate of Interest in the United States: A Quantitative Evaluation. IMF Working Paper No. 2022/030.